Where Would You Need to Go on Earth to See the Celestial Equator Very Near Your Horizon?

Reference Markers

Chapter index in this window —   — Chapter index in separate window

This material (including images) is copyrighted!. See my copyright notice for fair use practices.

Now for some reference makers: The stars rotate around the North and South Celestial Poles. These are the points in the sky directly above the geographic north and south pole, respectively. The Earth's axis of rotation intersects the celestial sphere at the celestial poles. The number of degrees the celestial pole is above the horizon is equal to the latitude of the observer. Fortunately, for those in the northern hemisphere, there is a fairly bright star real close to the North Celestial Pole (Polaris or the North star). Another important reference marker is the celestial equator: an imaginary circle around the sky directly above the Earth's equator. It is always 90 degrees from the poles. All the stars rotate in a path that is parallel to the celestial equator. The celestial equator intercepts the horizon at the points directly east and west anywhere on the Earth.

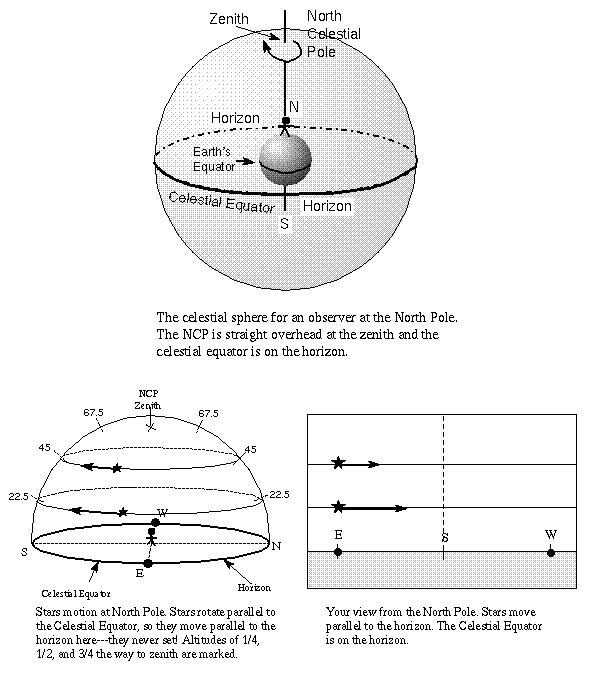
If you joined Santa last Christmas at the north pole (90 degrees latitude), you would have seen Polaris straight overhead and the celestial equator on your horizon. The point straight overhead on the celestial sphere for any observer is called the zenith and is always 90 degrees from the horizon. The arc that goes through the north point on the horizon, zenith, and south point on the horizon is called the meridian. The positions of the zenith and meridian with respect to the stars will change as the celestial sphere rotates and if the observer changes locations on the Earth, but those reference marks do not change with respect to the observer's horizon. Any celestial object crossing the meridian is at its highest altitude (distance from the horizon) during that night (or day). The angle the star paths make with respect to the horizon as they rise up or set down = 90 degrees minus the observer's latitude. At the north pole, the latitude = 90 degrees so the stars paths make an angle of 90 minus 90 degrees = 0 degrees with respect to the horizon---i.e., they move parallel to the horizon as shown in the figure above. For locations further south you will see in the figures below that the stars will rise up (and then set down) at steeper angles as you get closer to the equator.

During daylight, the meridian separates the morning and afternoon positions of the Sun. In the morning the Sun is ``ante meridiem'' (Latin for ``before meridian'') or east of the meridian, abbreviated ``a.m.''. At local noon the Sun is right on the meridian (the reason why this may not correspond to 12:00 on your clock is discussed a little later in this chapter). At local noon the Sun is due south for northern hemisphere observers and due north for southern hemisphere observers (though observers near the Earth's equator can see the local noon Sun due north or due south at different times of the year for reasons given in the next section). In the afternoon the Sun is ``post meridiem'' (Latin for ``after meridian'') or west of the meridian, abbreviated ``p.m.''.

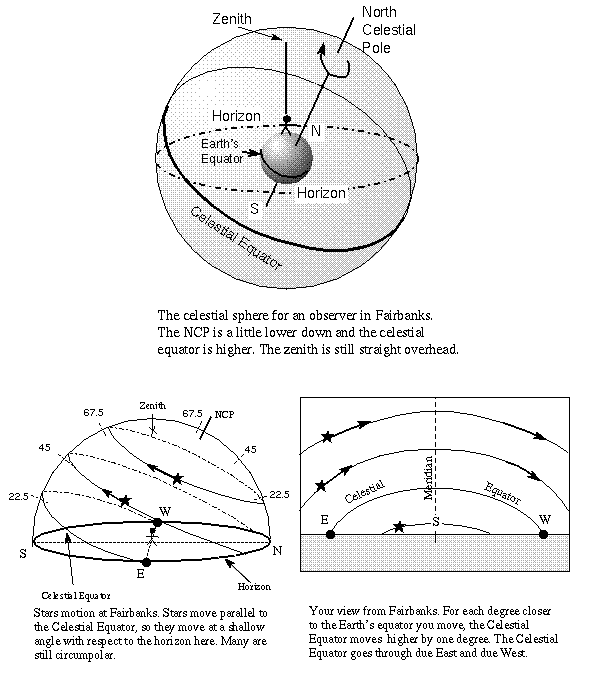
For each degree you move south with Santa in his sleigh, the North Celestial Pole (NCP from here on) moves 1 degree away from the zenith toward the north and the highest point of the celestial equator's curved path in the sky moves up one degree from the southern horizon. This effect has nothing to do with the distance between a celestial object or marker and you at different points on the Earth (remember that the celestial sphere has a practically infinite radius). In fact, observers on a spherical world only ten miles across would see the same effect! The picture above shows the celestial sphere for the far northern city of Fairbanks in Alaska. Since it is 25° south of the north pole, the NCP is 25° away from (north of) the zenith for Fairbanks observers.

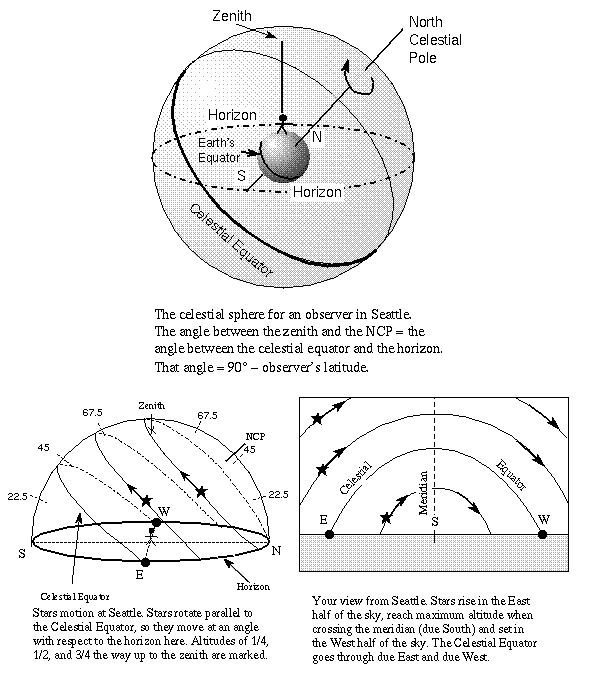
By the time you reach your hometown, the NCP has moved away from the zenith so it is now a number of degrees above the horizon equal to your latitude on the Earth. Remember that your position on the Earth is specified by a latitude and a longitude coordinate. The latitude is the number of degrees north or south of the Earth's equator. On a map or globe, lines of latitude run horizontally, parallel to the equator. The longitude is the number of degrees east or west of the 0° longitude line (the ``prime meridian'' on the Earth) that runs through Greenwich England. On a map or globe, lines of longitude run vertically, perpendicular to the equator. The celestial sphere for observers in Seattle and any other observer at the same latitude (47° N) on the Earth is shown above.

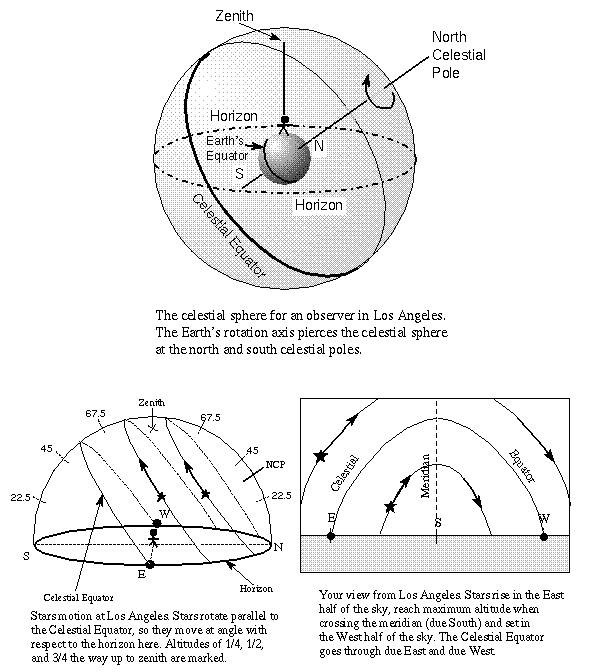
For another more detailed example, let's choose Los Angeles at latitude 34° N. The NCP is therefore 34 degrees above the north horizon. The diagram for latitude 34° N is shown above. Notice that finding the angle of the NCP above the horizon provides a very easy way of determining your latitude on the Earth (a fact used by navigators even today!). Because the Earth's equator is 90° away from the north pole, the celestial equator as seen in Los Angeles will arc up to 90 - 34 = 56 degrees above the southern horizon at the point it crosses the meridian. It still intercepts the horizon at the exactly east and west points. The stars rise in the east part of the sky, move in arcs parallel to the celestial equator reaching maximum altitude when they cross your meridian, and set in the west part of the sky. The star paths make an angle of 90 - 34 = 56 degrees with respect to the horizon.

If you are in the northern hemisphere, celestial objects north of the celestial equator are above the horizon for more than 12 hours because you see more than half of their total 24-hour path around you. Celestial objects on the celestial equator are up 12 hours and those south of the celestial equator are above the horizon for less than 12 hours because you see less than half of their total 24-hour path around you. The opposite is true if you are in the southern hemisphere.

Notice that stars closer to the NCP are above the horizon longer than those farther away from the NCP. Those stars within an angular distance from the NCP equal to the observer's latitude are above the horizon for 24 hours---they are circumpolar stars. Also, those stars close enough to the SCP (within a distance = observer's latitude) will never rise above the horizon. They are also called circumpolar stars.

To warm Rudolph's frozen nose, Santa heads down to the equator (0 degrees latitude). At the equator, you see the celestial equator arcing from exactly east to the zenith to exactly west. The NCP is on your northern horizon. At the equator you see one-half of every star's total 24-hour path around you so all stars are up for 12 hours. All of the stars rise and set perpendicular to the horizon (at an angle = 90 - 0 = 90 degrees).

Continuing southward you see the NCP disappear below the horizon and the SCP rise above the southern horizon one degree for every one degree of latitude south of the equator you go. The arc of the celestial equator moves to the north, but the arc still intercepts the horizon at the exactly east/west points.

Here is a summary of the positions of the celestial reference marks (note that ``altitude'' means the number of degrees above the horizon):

• Meridian always goes through due North, zenith, and due South points.
• Altitude of zenith = 90° (straight overhead) always.
• Altitude of celestial pole = observer's latitude. Observers in northern hemisphere see NCP; observers in southern hemisphere see SCP.
• Altitude of celestial equator on meridian = 90 minus the observer's latitude.
• Celestial equator always intercepts horizon at exactly East and exactly West points.
• Angle celestial equator (and any star path) makes with respect to the horizon = 90 minus the observer's latitude.
• Stars move parallel to the celestial equator.
• Circumpolar object's distance from celestial pole = observer's latitude.

Select this link to show an animation of the celestial sphere changing with latitude.

(You'll want your web browser to fill most of your screen!)

Select this link to show a Macromedia Flash animation of Orion star trails at different latitudes.

Check out the Rotating Sky module from the University of Nebraska-Lincoln's Astronomy Education program to explore the connection between your position on the Earth and the path of the stars (link will appear in a new window---choose the "Rotating Sky" part) and use their Rotating Sky Simulator in the Native Apps package (the Flash simulators no longer work with today's browsers).

Vocabulary

celestial equator	circumpolar	latitude
longitude	meridian	North Celestial Pole
South Celestial Pole	zenith

Review Questions

1. How do the positions of the celestial equator, celestial poles, zenith, and meridian depend on the latitude of the observer?
2. Would their position with respect to the horizon change if the Earth were only 200 miles in diameter? How about 80,000 miles in diameter? Why is that?
3. During a night, how do the stars move? What angle does their nightly path make with respect to the horizon? How does it depend on latitude?
4. What reference point is a celestial object on when it is at its highest position above the horizon?
5. Why do observers in the northern hemisphere see celestial objects above the celestial equator for more than 12 hours?
6. For northern hemisphere observers, which celestial object would be above the horizon for the greatest amount of time: one that is on the celestial equator, one that is 30° above the celestial equator, one that is 70° above the celestial equator, or one that is 40° below the celestial equator? Which one would be above the horizon the greatest amount of time for southern hemisphere observers? Explain your answer.

Go back to previous section -- Go to next section

Go to Astronomy Notes home

last updated: August 31, 2020

Is this page a copy of Strobel's Astronomy Notes?

---

Author of original content: Nick Strobel

Where Would You Need to Go on Earth to See the Celestial Equator Very Near Your Horizon?

Source: https://www.astronomynotes.com/nakedeye/s4.htm

Share this post